In order to increase understanding of our revered mechanical system, I'd like to calculate an energy budget for the recoil phase, that is, calc what the initial slide energy is and where it winds up. I'll measure energy in inch pounds, and the analysis will stop at frame impact.
It's often easier to analyse conserved quantities like energy or momentum rather than consider individual forces.
This is very rough so far, and these numbers could change a lot. I have an Excel sheet of this if there is a place to post it.
------------------------------------------------------
First calculate the initial slide energy,
Slide Assembly Initial Kinetic Energy = Bullet energy * (Bullet mass/Slide Assembly Mass) + Gas Effect = 368 ft lb * (0.033/1.15) * 12 (in lbs/ft lb) + 25 = 152 inch lbs
The first term I leave as an exercise (start with just E=.5 m v^2 and Momentum=mv for both slide and bullet), the second term is from Quickload for a fast powder.
Now calc how much of this winds up in the rec spring,
To Rec Spring = 2.2 in * 18.5 lbs. = 41 inch lbs
This was calculated by just multiplying the rating of the recoil spring by the compression. I'll do it properly by integrating the spring law when I get the spring coefficients , but iirc the spring rating is the load somewhere near the middle of travel, so this should be close. I could be remembering wrong here.
When the barrel unlocks and hits, all of its energy goes into heat or slight deformation,
Lost when barrel links down and hits = 27 inch lbs
Took the barrel mass as a fraction of slide assembly mass to calc this
It takes some energy to cock the hammer,
Hammer Cocking Energy = 8 inch lbs
Did a dynamic analysis by eyeballing the radius of gyration of a traditional hammer and applying some rotational equations. This one is a bit complicated, but the result of 8 inch pounds is consistent with energy changes observed when modifying the FPS.
Ejection work = 2 inch lbs
Wild guess
Other Losses = 17 inch lbs
Frame Impact = 57 inch lbs
Extraction Work=0
Using niemi's data for slide movement, we now get a frictional dissipation and other loss of for his setup as 11 inch pounds. I am scaling this 10 17 for a full load.
Among our assumptions here is that other losses are the same the same for differnt slide velocities.
Some energy must go into the frame and shooter before frame impact, but the Tripp video doesn't show much of this.
CA... you're makin' this thing way yonder more complicated than it needs to be.
I assume that you're attempting to show that nothing changes regardless of firing pin stop radius.
There are much simpler ways to determine what difference the 1/16th radius makes.
Ways that don't require a calculator with full scientific functions. A 16 year-old 1st year Algebra student could do it with a pencil and paper.
He's not attempting to show nothing changes, he's attempting to understand what does change, and why.
Quote:
he's attempting to understand what does change, and why
Yep...and the "What/How Much" can be determined in about 10 minutes.
Hint:
It would be pretty much a direct proportion.
We already know why. There's only one change made in the system.
This thing ain't exactly a Swiss watch. It was designed by a man who never had any sort of formal engineer skoolin'.
Quote:
Some energy must go into the frame and shooter before frame impact, but the
Tripp video doesn't show much of this.
I think you will find this to be a pretty important part of the effect.
Consider two extreme cases: slide locked to frame, and slide free to move with no hammer to cock and no recoil spring.
In the first, slide energy is maximized, and frame energy is zero until impact.
In the second, slide energy is minimized, and the frame starts absorbing energy right away.
The total energy absorbed by the gun is smaller in the second case, because the recoiling mass is higher (slide plus frame, vs. just slide).
In real life, the interaction is somewhere in between these two extremes. The effect of the small-radius FPS is to move you slightly towards the locked- slide end of the continuum (compared to the large-radius FPS); slightly decreasing slide energy by getting the frame involved to a greater degree before the bullet leaves.
Or course, felt recoil also depends on how the frame push is distributed in time, and spreading it out a bit by doing a bit more early and a bit less late probably gives a decreased sensation compared with a sharp jolt. Plus the moment of inertial of the system is higher when the slide is forward, so there's less tendency for the muzzle to flip upward sharply.
Quote:
Consider two extreme cases: slide locked to frame...
First... the slide is never "locked" to the frame. That would turn the gun into a fixed breech weapon... like a revolver. The slide /barrel assembly IS the gun. The frame is no more than a gun mount. The gun recoils. The gun is mounted on rails. The frame only limits its travel.
Quote:
Or course, felt recoil also depends on how the frame push is distributed in
time
That's exactly what the small radiused firing pin stop is all abou, and how it affects the entire recoil sequence. Time and distance and momentum.
Quote:
First...the slide is never "locked" to the frame.
Sure, but I'm just suggesting that as a thought experiment, one can look at the locked case as one extreme of behavior, and the free-motion case (which also never occurs, unless your hammer spur and recoil spring plug both give out at the same instant) as the other extreme, and know that, in real life, you have to be somewhere in between those two in terms of slide energy. CA is building an energy budget here, and I'm suggesting he start by putting limits on his range and work inward from them. It's sort of a perturbation analysis: solve the easy case, add in hard stuff to that result.
As an aside, I have an EGW stop sitting in my "gun stuff" pile, waiting to be fitted. Preliminary measurements indicate something like .01 inches of material need to come off, which is going to be a pain with my very limited file collection. Should get to try it early next month. I'm hoping it changes things enough to convince my wife to get an 1911, which would give me the necessary budget for either another RIA or a budget build.
Quote:
Or course, felt recoil also depends on how the frame push is distributed in
time, and spreading it out a bit by doing a bit more early and a bit less late
probably gives a decreased sensation compared with a sharp jolt.
Nice thought experiment, RobL. The Tripp video does not show much frame movement before impact, and the frame does not appear to be braced horizontally. The wooden pillar which the hand is on looks quite wobbly. But the fact that limp wristing can make a difference in slide movement (relative to the frame) indicates that energy transfer to the frame can be important.
But using niemi's data there are only 11 inch pounds unaccounted for, and he was using the FPS mod.
I'll start calling "Friction" "Other Losses" which would include this. But I think we're pretty close to the free frame side.
As far as the FPS mod effectiveness goes, I've been thinking along the same lines you have. But my primary goal in making this energy budget is not to analyse a change in something as unmeasureable as felt recoil.
What I want to do is understand the energetics so I can determine the size of the energy reserve against dissipation increases (such as increased friction due to foreign debris) and load variations.
Tuner, your bumblebee example does indeed show that simple models can lead one astray, and that we should be skeptical of theory. I'm with you there. I always ask my students to be as critical of their own work as if it were done by their worst enemy, who is competing with them for promotion and significant other
Btw, when you say that the fps mod effect can be reduced to a simple proportion, which quantity is proportional to which in your view, and do you have an estimate for the constant of proportionality?
RonL, I think you're on to something with your frame movement idea. I once built a .38 Super on an Al officer's frame, and just the change in frame mass made the pistol very sensitive to limp wristing when the mag was getting light on the last few rounds.
It seems like we could model part of this effect as proportional to the intial slide velocity (for sliding friction), and part as proportional to the rec spring strength (for energy losses to the frame via the rec spring). I'll put in the cocking energy also, weighting the above effects 1,1,.5.
Niemi used a pretty standard setup so I'll still use his data to calibrate. This should reduce the very large frame impact above by a bit.
ETA - But remember that my initial calculations in the change in slide velocity caused by the fps mod used the observed change in recoil spring weight, and not the model in this budget. Frame losses would be included in this.
And actually it might be best to model this effect as proportional to the square of the quantities above.
We're getting some insights.
I can't help on the mathematical model you are trying to come up with, but the experiment that proved the validity of the square-bottom FPS is a very simple one. Go to a door in your house and try closing it by pushing it with your finger, near the door knob. The try the same thing by pushing the door with your finger, near the hinges. See the difference?
Quote:
I can't help on the mathematical model you are trying to come up with, but
the experiment that proved the validity of the square-bottom FPS is a very
simple one. Go to a door in your house and try closing it by pushing it with
your finger, near the door knob. The try the same thing by pushing the door
with your finger, near the hinges. See the difference?
Very good illustration John.. But I think in the case of the 1911.. It might be so much force pushing that (door) that it really doesn't matter where on the dooy you push it from..
Although I for one do feel the difference the EGW FPS gives... So, in the end your illustration might be spot on...
There's a difference in the required force to achieve the same acceleration, but there's no difference in the total energy required to move the door from one position to the next (to a first approximation; if you push really hard, you might affect the friction of the hinges). Tuner has stated that shok-buffs in EGW guns last longer than in "standard" guns, meaning the slide is actually absorbing less energy. That is the reason why I believe the frame must be picking up part of the energy and momentum before the bullet leaves the barrel, making the gun more "locked" than the large-radius case.
In order to increase understanding of our revered mechanical system, I'd like to calculate an energy budget for the recoil phase, that is, calc what the initial slide energy is and where it winds up. I'll measure energy in inch pounds, and the analysis will stop at frame impact.
It's often easier to analyse conserved quantities like energy or momentum rather than consider individual forces.
This is very rough so far, and these numbers could change a lot. I have an Excel sheet of this if there is a place to post it.
------------------------------------------------------
First calculate the initial slide energy,
Slide Assembly Initial Kinetic Energy = Bullet energy * (Bullet mass/Slide Assembly Mass) + Gas Effect = 368 ft lb * (0.033/1.15) * 12 (in lbs/ft lb) + 25 = 152 inch lbs
The first term I leave as an exercise (start with just E=.5 m v^2 and Momentum=mv for both slide and bullet), the second term is from Quickload for a fast powder.
Now calc how much of this winds up in the rec spring,
To Rec Spring = 2.2 in * 18.5 lbs. = 41 inch lbs
This was calculated by just multiplying the rating of the recoil spring by the compression. I'll do it properly by integrating the spring law when I get the spring coefficients , but iirc the spring rating is the load somewhere near the middle of travel, so this should be close. I could be remembering wrong here.
When the barrel unlocks and hits, all of its energy goes into heat or slight deformation,
Lost when barrel links down and hits = 27 inch lbs
Took the barrel mass as a fraction of slide assembly mass to calc this
It takes some energy to cock the hammer,
Hammer Cocking Energy = 8 inch lbs
Did a dynamic analysis by eyeballing the radius of gyration of a traditional hammer and applying some rotational equations. This one is a bit complicated, but the result of 8 inch pounds is consistent with energy changes observed when modifying the FPS.
Ejection work = 2 inch lbs
Wild guess
Other Losses = 22 inch lbs
Frame Impact = 52 inch lbs
Extraction Work=0
Using niemi's data for slide movement, we now get a frictional dissipation and other loss of for his setup as 11 inch pounds. I am scaling this to 22 for a full load with a 18.5 lb spring and a higher slide velocity.
Among our assumptions here is that other losses are the same the same for different slide velocities.
Some energy must go into the frame and shooter before frame impact, but the Tripp video doesn't show much of this.
Quote:
Some energy must go into the frame and shooter before frame impact, but the
Tripp video doesn't show much of this.
If the frame/shooters grip apparant mass or stiffness was very large compared to the slide/barrel it wouldn't show much movement despite accounting for a lot of energy. Small changes would have a large effect on the result.
There isn't any question the shooters mass is much larger than the entire weapon, the only issue is the stiffness of the connection.
My take on the slide stop modification is it actually transfers more recoil earlier to the shooter. If there was no hammer, the barrel and slide could only transfer force to the shooter through the recoil spring and friction. Adding the hammer is adding a lot as anyone who's racked both a cocked and uncocked 1911 will know. Adding the short radius fps increases the racking effort eary on, but it ends sooner. Same energy to the hammer spring, but the timing and peak force are different.
Instead of a thought experiment, try a real one. Start with some midrange reloads with 230 gr ball using a fast powder like Red Dot. Back off the load in 0.2 gr increments. Shoot one round at a time and note the powder charge when the slide fails to lock back, ejection problems start, and when the empty doesn't leave the chamber. Repeat with a short radius FPS, and then a no radius FPS.
The shorter the radius, the more powder (energy) it takes to run the gun. Something is changing.
This sounds like an excellent experiment. In recent posts in the fps mod thread, niemi discussed his device that measured slide travel by the scraping of sight black. He used light loads and measured slide travel with the fps modded, but not also with a stock fps. This method could also be used to compare.
In a way the experiment has already been done, since Tuner says that a change from a 16 lb rec spring to a 14 lb one compensates for the fps mod (I presume he used ejection characteristics as the benchmark). This makes a diff in slide energy at ejection of about 3 inch pounds, which is about 2% of total slide energy, which gives a change in slide velocity of about 1%.
Quote:
If the frame/shooters grip apparant mass or stiffness was very large
compared to the slide/barrel it wouldn't show much movement despite accounting
for a lot of energy. Small changes would have a large effect on the
result.
Here is my logic on the issue of energy transfer to the shooter.
The work done on a body by a force (the energy transfer through contact forces in our case) is equal to Force * Distance regardless of the mass of the body. If I push on the entire Earth with a force of 20 lbs, and the Earth moves 2 feet, I have still only given it 40 ft lbs of energy. Of course it would take a long, long time to move the Earth 2 feet with this force, and the resulting velocity would be small, to say the least. This is why it works out.
In the Tripp video, the frame moves back about 10 pixels from ignition to frame impact (I have been analysing the close up, but admittedly have had trouble getting consistent results). I have calibrated the pixels at about .00067 feet using the image of the case mouth which doesn't appear to have too much cosine effect due to tilt. This means that the frame moves back about .080" Five pixels of this occurs suddenly near the beginning of extraction (near the time of barrel/frame bed impact). I have no explanation for this.
Anyway, even if the frame exerted a force of 50 lb. on the shooter's hand for this entire travel we would still only have an energy transfer of 4 inch lbs, and I can't see where 50 lbs. would come from over the whole recoil cycle.
The largest frame velocity shown during the sudden motion mentioned above is about 1/7 of slide velocity, which would of course lead to an energy of around 1/50 of the slide energy times the ratio of frame/slide mass. This comes out to very roughly 4 inch lbs by my model.
I have nothing against the fps mod, I hope it works as well for me as it seems to for some (and I'm going to try niemi's fps bevel, what the heck). And if there's something wrong with my model or the logic behind it, I certainly want to discover it.
Of course the bumblebee thing is exaggerated, weren't the analysts using some simple model of wing loading or energy consumption or something? But it does make the point that hard data trumps the models. I just wish we had more of it. I wish we had high speed video from all angles including extreme close ups looking into the port, at 5 times the Tripp rate and 5 times the resolution, with easily readable scales on all parts and all of the data about the pistol given.
And while I'm dreaming, the above with both kinds of firing pin stops, and both kinds of mag lips.
Unless I missed something... the rocket scientists have failed to consider one small, but important point. They've been concentrating so hard on springs and leverage and such... that they missed the obvious.
Clew:
Felt recoil in an autopistol comes from the springs compressing and the slide's impact with the frame.
The more resistant the mainspring/hammer function... the more immediate recoil will be transferred to the shooter's hand because it begins to function more like a fixed-breech weapon. Likewise for the recoil spring. This impulse is over quickly, and adds little to the overall effect of the rest of the recoil phase.
Clew 2. The pistol is recoil operated. What generates the force needed to operate the pistol? When is the force available to operate the pistol?
Quote:
The more resistant the mainspring/hammer function... the more immediate
recoil will be transferred to the shooter's hand because it begins to function
more like a fixed-breech weapon.
That is, I believe, the very point I have been making in several of these threads for months.
Quote:
That is, I believe, the very point I have been making in several of these
threads for months.
Then you should be able to find the quarter horse in the Zebra herd.
Okay. Last clew.
The Recoil Operated 1911 is almost... ALMOST... a delayed blowback design.
Delayed being the operative word.
Quote:
Delayed being the operative word.
Does the delay come from the time it takes the case of ignited cartridge to move aft and contact the breechface?
Got no idea how time it takes, but the distance in the average gun is 0.009" or 0.016" (depending on whether Ordnance or SAAMI chamber data is used).
There's also the delay after this one between the time the case head hits the breechface (and starts pushing the slide aftward over the barrel) and time when the horizontal 0.0125" gap between the locking lugs gets closed - and the barrel starts going back too.
Are either of these the delays you hinted about, or did I merely chase up a couple of zebras?
Quote:
Unless I missed something... the rocket scientists have failed to consider
one small, but important point. They've been concentrating so hard on springs
and leverage and such... that they missed the obvious.
For a guy that disparages engineers, you're not a bad one. JMB many not have had a formal engineering education (anybody have a good biography reference?), but that he was an applied engineering genius is a matter of historical record.
The answer to Clew 2 is the same force that is driving the bullet. The when is over before the barrel and slide unlock, or the first .2" or so.
I like to hang numbers on these things because it often comes down to a sense of scale. After CAs last post, I tried another experiment. I took my wifes 5" para thats been slicked up as much as I can to reduce friction and tested it on a digital scale. After subtracting the weight of the gun, it took 9 pounds force to bring the slide half was through the unlocking phase or ~0.1" when the hammer was cocked using a fairly fresh 16# recoil spring. With the hammer down, it took 13 pounds force using a fps with a very generous radius. Changing the fps to an EGW with a square edge increased the cocking requirement to 17 pounds force.
So it looks like about 9/13 or 70% of the available recoil energy is transfered to the slide with a large radius fps. A no radius fps drops this to 9/17 or 53%. The energy tranfered to your hand goes up .7/.5 or 140%. A small radius would give an effect somwhere in between.
The small radius stop actually transfers more of the available recoil energy to the hand. But it starts sooner and is acting through a spring instead of going "clack" on the VIS or slide stop pin.
If we change the recoil spring, we have to pick our poison. Less of a clack on the VIS gives more on the slide stop pin, and visa versa.
The problem is the amount of energy available to run things is fixed, if more is going into the frame and hand, less is available to finish the ejection and rechambering. The envelope that the gun will operate under is narrower with a small radius stop.
I've been putting a large radius on my EGW stops since a no radius stop turned one of my 3" guns into a fixed breech weapon with practice loads it had previously cycled.
The EGW fps is in every one of my guns and most of the ones I've worked on simply because it solves so many other problems cheaply and for good.
Isn't the friction of the bullet moving down the bore trying to pull the barrel forward while the expanding gases that push the bullet are trying to push the case against the slide but can't do too much till the expanding pressures diminish to release the case against the chamber?
I don't know what the rearward and downward release of the barrel from the slide plays in the delay but seems it's got something to do with some'ent.
You're gettin' close, artie. Mighty close.
I don't disparage engineers. I was raised by one. He was also able to think outside the box... and realized that engineering is more than formulae, theorums, and slide rules. He said that some engineers are fun to watch... like ants tryin' to climb out of a glass jar that they worked hard to climb into.
One of the greatest lessons that he taught me was how to think outside the box before gettin' in it.
John Browning didn't have an engineering degree, and was actually quite proud of it.
He felt that it got in the way sometimes.
Quote:
I don't disparage engineers. I was raised by one.
You're father must have been a pretty good engineer, it doesn't occur the good ones that there is a box. The sense of scale that allows them to see the forest for the trees is another important characteristic. The percentage of good to bad is probably the same as any other profession, the difference with engineers is they generally get promoted to further incompetence as managers or what not before they've finished their appreticeships as engineers.
Is the remaining bit of your analysis the fact that the 1911 is really a blow back design? Except that it exploits the barrel weight and not just a bolt. Sorta, because the combined weight is locked long enough to contain the cartridge until the pressure drops. The combined weight buys the time to lower the locking forces long enough that the puny link can reliably unlock it.
The bulk of the bullet acceleration happens in the first inch or so, and the acceleration decays quickly after that, so anything that further delays the unlocking further reduces the energy put into the slide. Since the energy has to go somewhere, enhancing the "fixed breech" effect puts even more of if into the grip. Again, through the springs and not a clack on the VIS or slide stop pin.
Quote:
The bulk of the bullet acceleration happens in the first inch or so, and
the acceleration decays quickly after that, so anything that further delays
the unlocking further reduces the energy put into the slide.
I am not an engineer, but I need to ask:
If the force pushing the bullet forward is acting as long as the bullet is in the barrel, and that force is constant (if not increasing, since pressure is continuously rising inside it) how can the bullet's acceleration decay after the first inch of travel? With my limited physics knowledge, as long as the force is being applied to the bullet acceleration is constant (if not increasing).
Acceleration stops when the force stops, which is when the bullet leaves the barrel.
Am I missing something here?
Quote:
With the hammer down, it took 13 pounds force using a fps with a very
generous radius. Changing the fps to an EGW with a square edge increased the
cocking requirement to 17 pounds force.
I'm glad that more folks are making measurements, but there's a bit of physics here which is quite important to know.
You can get some insight if you compare the words in color, and remember that Work = Energy Transfer (in our case) = Force * Distance
Tuner, you too have conflated these two quantities in previous posts, and the distinction is essential for understanding what is going on here.
The lower cam lobe of the reduced radius fps makes for a higher cocking force, but exerts this force through a smaller distance. In the static, slow- racking-of-the-slide case, the cocking energy will be the same for the two radii.
In the dynamic case of fast cocking, the kinetic energy of the hammer can become important. This is the whiplash effect (to use niemi's words) discussed in the other thread. Niemi's experiment suggests that this is important for the modded fps. This appears to be true with the stock fps also. A careful viewing of the Tripp video shows the hammer overcocking and bouncing against the center rail at least twice. And that was a lightened hammer. A traditional hammer with its much higher rotational inertia would increase this effect.
This does increase the cocking energy, and does increase the energy transfer to the frame during this phase, as has been discussed by many folks in both threads.
This gives us a *testable* prediction, by the way, that a lightened hammer will bleed less slide energy than a traditional one, even though the slow cocking force might be the same. This means that a different hammer design could have as much effect or more as changing the slide stop radius. This also means that the radius mod would have less effect with the lightened hammer.
The test would be to use niemi's slide travel measuring setup to get a precise idea of slide energy changes.
Intuition has a vitally important role in these problems, both for engineers and others, but to place faith in it to the point of certainty is a mistake. The same goes for mathematical models. Hard data rules here, and we are very short on it.
Quote:
Am I missing something here?
Two things: I didn't take "acceleration decays" to mean "decelerates." That is, the bullet is still accelerating, just not as much as before, because the force (pressure) has dropped.
The other thing is that friction with the barrel is also acting on the bullet; if the barrel were long enough to let pressure drop to where the force on the bullet's base was less than the force from friction, it could actually decelerate. That's only going to happen with a squib.
The pressure has dropped? Before the bullet exiting the barrel?
Friction is acting on the bullet from the moment it starts moving. Of course, given that friction depends on velocity (if memory serves me right), it increases with the speed of the bullet, but this requires some more in-depth study before being able to say that the bullet's acceleration decreases while the bullet is still in the barrel. There are too many variables here, the rate of combustion of the powder is a significant variable. Are the gases generated instantly or over a period of time? And if they are not generated instantly, is the rate of gas generation linear with time or not? And for how long? etc. etc. etc.
As I said, I am no engineer and I have not studied the particular phenomena in any significant depth, I am just wondering.
I'll go ahead and do this at the risk of spoilin' all the fun...
I'll try to keep it as short as possible.
I know that (much of the) the following is a given. I use it because it's leading up to something.
Neimi... There are several things that delay the slide. Anything that CAN act as an outside force WILL be an outside force.... even a fruitfly standing on the rear sight at the moment of acceleration. Anything that has the opportunity to delay it will delay it.
Artie... The slide moves .1 inch nominally when the linkdown phase begins. The bullet must be gone at or before that point, because the link can't move the barrel vertically while the system is under pressure horizontally without damaging the lugs and the link.
The majority of the acceleration occurs in a half-inch or less, depending on powder burn rate. Peak acceleration coincides with peak pressure... which means peak force.
BUT... As long as the bullet is present and accelerating, the slide is being pushed. Once the bullet is gone, force on the slide is also gone. Any movement after that point is all momentum... bullet and slide.
The dwell time of the bullet depends on its rate of acceleration... regardless of how far the slide has moved. Barrel linkdown is a timed event. It will happen where it's designed to happen. The bullet is the variable here.
Any slide delay before the bullet exits has a greater effect on the slide's speed and momentum than any outside force that is applied AFTER the bullet's exit. The bulk of the delay occurs before the bullet exits. The whole point of delay is to give the bullet time to leave the system so that pressure can drop to safe levels. Any delay after that is incidental.
The single largest delaying force for the slide is the bullet itself. The second most important one is the hammer and mainspring assembly. The recoil spring has very little delaying effect. Its main function is in returning the slide to battery, though a strong enough recoil spring can prevent the slide from cycling at all... but that would damage the spring plug, barrel bushing, and the thin bushing lug raceway in the slide.
If we assume that two rounds of ammunition will provide exactly the same rate of acceleration in two hypothetcally identical pistols... one equipped with the standard 7/32nds radius FP stop... and the other with a 1/16th radius stop...the one with the smaller radius will provide a very slight but very signifigant delay while the bullet is hurtling toward the muzzle.
Let's assume that both pistols time the beginning of the linkdown phase at .100 inch of slide travel.
Let's assume that the bullet will exit at .098 inch of slide travel in pistol A... with the 7/32nds stop radius.
By means of lesser mechanical advantage, Pistol B's smaller stop radius will delay the slide for a split fraction of a fraction second longer... so that the slide has only moved about .070 inch.
.100 inch of slide travel will move the hammer far enough to engage the half- cock notch. That's the breakover point for the hammer... like a compound bow.
At .070 inch of slide movement... the hammer hasn't reached the break point. The mainspring is still fighting it... and the bullet is gone... so there isn't any more force available to drive the slide. While it's true that there isn't much acceleration after peak pressure/force... there is some... and everything means something.
So... decreasing the radius on the stop has affected several changes to the system... not just one. It delays the slide, and degrades part of the slide's momentum, transferring it to the grip. That effects a change in the recoil's direction... from horizontally to almost vertically.
It delays the slide at the right instant... before the hammer has reached the breakover point, and after any residual force on the slide is no longer available to "help" the slide overcome the mainspring. This tends to multiply the effect of the small radius beyond what added force is required to hand- cycle the slide.
It's been calculated that a 1% additional force is required... but hand- cycling suggests that it requires more than that. I've never measured it, but it would be fairly easy to determine with a simple fish scale. Remember that the reason for the change in 1918 was that the mounted troops complained that the gun was too hard to hand-cycle with the hammer down... but were quite happy with the reduced effort provided by the 7/32nds stop. I seriously doubt that young, strong men would be able to detect a 1% difference.
So... The effect of the small radius stop is more than mere effort alone. It's about time and timing, too.
Remember the engineer's dictum:
"When you make one change in the system, it affects several things within the system."
There is no such thing as "Just one little change."
I can almost hear the electric pencil sharpeners buzzin'.
Quote:
The pressure has dropped? Before the bullet exiting the barrel?
Yes.
Peak pressure occurs early in the bullet's flight. Pistol powders peak rapidly and violently... and pressure drops dramatically after the peak.
The reason that acceleration continues after the peak is due to Newton 1B:
"Objects in motion tend to remain in motion."
It requires less force to keep an object moving than it does to get it moving in the first place... and it requires less force to accelerate it than it took to get it up to speed at the instant of peak pressure.. .and the faster it's moving, the easier it becomes to accelerate.
By "Decay" I think artie was referring to accelerating force. In these techincal explanations, it's often easy to fail to use precisely the right words. One gets caught up in something that he understands perfectly, but just doesn't convey it clearly. That's why teaching is such a tough job.
Quote:
The other thing is that friction with the barrel is also acting on the
bullet; if the barrel were long enough to let pressure drop to where the force
on the bullet's base was less than the force from friction, it could actually
decelerate. That's only going to happen with a squib.
Correct... up to the point about only happening with a squib.
Several years ago, a gun writer/gunsmith conducted an experiment with rifles. He wanted to show the differences in muzzle velocity with different barrel lengths.
He started with a 26-inch barrel... a 700 Remington, I think... and fired several rounds with different powders... lopping off an inch at a time and refiring each lot.
He discovered that with... certain powders... he actually was able to get higher velocities with shorter barrel lengths. This suggested that the quick powders started to run out of steam at a certain length, and the bullet was decelerating due to friction... but not quickly enough to bring it to a stop.
Back in the day we called the first derivative of acceleration, "Jerk."
Also back in the day the rule of thumb for .22LR was that maximum MV was attained with a 16" barrel.
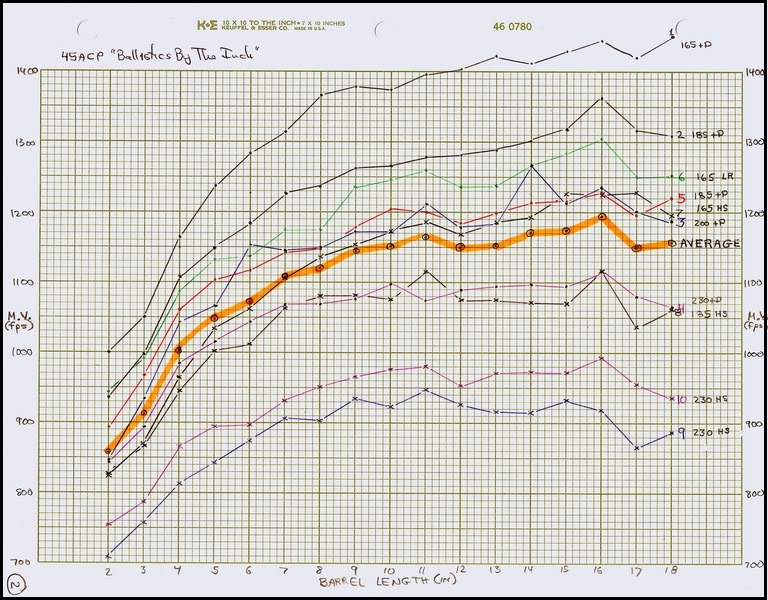
The guys at "ballistics by the inch" found that 230-gr Federal .45ACP peaked at around 10" barrel length. Of course, your mileage will vary depending on your particular barrel and specific .45ACP cartridge employed.
Quote:
The guys at "ballistics by the inch" found that 230-gr Federal .45ACP
peaked at around 10" barrel length. Of course, your mileage will vary
depending on your particular barrel and specific .45ACP cartridge
employed.
Walt... Precisely.
Anticipating the agruments over bullet-induced delay. May go ahead and write up the response ahead of time while I've got a few free moments this AM.
Quote:
He started with a 26-inch barrel
Fair point, but I was thinking of our beloved 5" .45 ACP. No way you're dropping pressure enough to slow it down unless you've seriously undercharged it.
Let's revisit the 1% difference calculation for a minute.
As noted... I don't think that a 1% difference... plus or minus... would be noticed by young men. I know that in lifting a weight off the ground, I can't tell the difference between a 100-pound weight and a 101 pound weight... and I don't believe that anyone else can, either. In fact... a young man in relatively good shape and of average strength would be hard-pressed to determine the difference between 100 and 110 pounds, given equal acceleration rates for both.
But hand-cycling doesn't tell the whole story. There's a big difference in dynamics when something is operated slowly, and when it's cycled at high high speed...as in during the normal functioning of the gun.
Resistances that were easy to overcome when the cycle was slow suddenly become major obstacles when the speeds take a sharp rise. Lifting a 100-pound weight three feet off the ground at a rate of one foot per second is pretty easy. When the rate of acceleration goes to 100 feet per second... it becomes a whole new ball game. The mass remains constant, but the force requirement goes out the roof.
So... Assuming that the smaller radius on the stop does in fact increase the force requirement by only 1%... the speeds at which that added 1% is imposed makes it a much different critter.
Small changes to the system very often create large effects.
Quote:
The guys at "ballistics by the inch" found that 230-gr Federal .45ACP
peaked at around 10" barrel length.
Other cartridges, however, seemed to peak at barrel lengths about twice that long:

But the 1911's not a Thompson Contender as was used in Ballistics By The Inch. Which begs this question:
How long can a 1911 barrel be before linkdown starts with the bullet still in the barrel (and the lugs still pressure-locked)?
Quote:
Other cartridges, however, seemed to peak at barrel lengths about twice
that long:
Depends on the powder burn rate and the leade. With a quick powder like Bullseye or HP-38 and a short leade with an abrupt transition to full rifling depth... it can peak before the bullet clears the case.
Quote:
How long can a 1911 barrel be before linkdown starts with the bullet still
in the barrel (and the lugs still pressure-locked)?
Don't know. Longslide guns ain't my cuppa tea, so I never really thought about it very much. One thing is for sure. The barrel ain't gonna link down while the lugs are engaged under pressure without some damage.
Quote:
The barrel ain't gonna link down while the lugs are engaged under pressure
without some damage.
Guess I got to wondering because someone had posted a pic of a 1911 barrel that was, IIRC, 12" long a year or so ago!
Sounded to me like a good way to bust a link and bend a slide stop pin - or worse.
Quote:
The mass remains constant, but the force requirement goes out the roof.
Yep, that's Work; Work = Force x Distance / time. Work can also be related to changes in energy. For the clean & jerk, energy will be the kinetic energy plus the potential energy. Do it faster, and the KE goes up by the square of the speed, while PE is invariant at any given height (basically it's the height from the, in this case, ground of the mass, under the influence of gravity).
Quote:
Yep, that's Work; Work = Force x Distance / time. Work can also be related
to changes in energy. For the clean & jerk, energy will be the kinetic energy
plus the potential energy. Do it faster, and the KE goes up by the square of
the speed,
And that, my good sir, is the horse that's been standin' in the middle of the zebra herd the whole time.
Well...One of'em, anyway.
Kudos, Walt.
Quote:
Guess I got to wondering because someone had posted a pic of a 1911 barrel
that was, IIRC, 12" long a year or so ago!
Well... You've had a few days to study on it, Dan. Still haven't found the horse?
Back to the original subject, does not the pressure decrease as the bullet moves down the barrel due to the increased volume? Or is the powder still producing thrust to the point of the bullet exiting the barrel?
Quote:
Back to the original subject, does not the pressure decrease as the bullet
moves down the barrel due to the increased volume?
Yes.
Quote:
Or is the powder still producing thrust to the point of the bullet exiting
the barrel?
As long as the bullet is present... thrust will be applied to the system. It won't be nearly as much as when pressure and force was at its peak... but it will still push.
Quote:
Back to the original subject, does not the pressure decrease as the bullet
moves down the barrel due to the increased volume? Or is the powder still
producing thrust to the point of the bullet exiting the barrel?
According to Boyle's Law, as the volume increases, the pressure goes down - for a fixed volume of gas a constant temperature. If the exothermic chemical reaction is ongoing, the temperature as well as the mass of the gas is most likely still increasing; if the increase in temperature offsets the increase in volume, the pressure will maintain or increase as the case may be. (We neglect here the effect on temperature of increasing the volume of a fixed mass of gas, which is how refrigeration works.
Boyle's Law in effect. Plug in an event that lasts for a few nanoseconds, and the dynamics change. While there's still a chemical reaction/heat occurring... the time frame is so brief, that its winndow of opportunity gets pretty narrow. Like popping your finger on and off of an electric eye. You don't get burned because of the dwell time.
When a barrel gets hot, it's not the temperatures of the burning powder that contributes to that as much as it is bullet/barrel friction. If somebody could create a steel alloy that cut friction to 1% that of ordnance steel... not only would velocities go out the roof, but peak pressures would drop, and barrels wouldn't heat up for dozens or even hundreds of rounds downrange.
Quote:
Back to the original subject, does not the pressure decrease as the bullet
moves down the barrel due to the increased volume?
According to this http://yarchive.net/gun/pistol/1911_barrel_length.html peak pressure is reached after the bullet has moved 0.3". And for a round of GI Ball, that's when the base of the bullet is at the case mouth.
But even though the pressure subsides after reaching its peak, it's still enough to overcome the friction of the bullet in the barrel - so the bullet continues to accelerate all during its remaining 4" trip down the barrel. The amount of acceleration decreases, however. At least that's my understanding.
Niemi... Yep.
Ever spot the horse in your long barrel linkdown question?
The 12-inch barrel has a lot more mass than the 5-inch does. Adding mass to the reciprocating assembly delays and slows it down... giving the bullet more time to exit.
Quote:
It's been calculated that a 1% additional force is required...but hand-
cycling suggests that it requires more than that. I've never measured it, but
it would be fairly easy to determine with a simple fish scale.
Tuner, you're conflating force and velocity again. The calculation suggested that the fps mod would make 1% distance in slide velocity, which came from a 2% difference in slide energy (I'm rounding). The equation is for our situation is
Work = (Change in) Kinetic Energy = Force * Distance
One can't measure Work with a fish scale alone, one also needs a ruler to get a distance to multiply by.
And sometimes intuition can be refined by *measuring*. That generates data which can be used for lots of things later.
Quote:
So... Assuming that the smaller radius on the stop does in fact increase
the force requirement by only 1%... the speeds at which that added 1% is
imposed makes it a much different critter.
The speed at which a body is moving does not change the equation
Work = (Change in) Kinetic Energy = Force * Distance
In a way, the speed is already in there since Distance = Velocity * Time
Quote:
Yep, that's Work; Work = Force x Distance / time.
I'm glad we're getting more physics into this (the Boyle's Law reference was spot on) but I have to say that there are stripes on the above. Time isn't a factor in the Work equation, you're thinking of Power.
Quote:
The 12-inch barrel has a lot more mass than the 5-inch does. Adding mass to
the reciprocating assembly delays and slows it down...giving the bullet more
time to exit.
Gad, I hadn't even got close!
But (while conjuring up zebras) I was struck by how close to being equal the FPS/hammer face bottom gap and the gap between the slide & barrel lug faces are when a GI gun's in battery.
The 0.0125" lug face gap allows the pressurized case to accelerate the slide back without the barrel before the gap is closed and the slide starts dragging the barrel along with it.
At about that same distance (and time), the FPS/hammer face bottom gap gets closed and the hammer gets "flicked" back out of the way - effectively eliminating any further affect it may have on the retreating slide/barrel assembly.
In short: when the slide picks up the additional burden of the barrel it gets the hammer (and mainspring) out of the picture.
Wonder if JMB made it that way, or is it just a coincidence?
Cheers
P.S.: FWIW, the barrel in the gun used in all those pix had a barrel with a lug face gap (endshake) of about 0.005".
Quote:
The 0.0125" lug face gap allows the pressurized case to accelerate the
slide back without the barrel before the gap is closed and the slide starts
dragging the barrel along with it.
A couple more horses hidin' amongst the zebras there.
First... Since the slide hits the barrel hood, it may not leave the full .012 gap in the lugs.
Second... If the hood to breechface fit is tight...that gap doesn't exist... or there's not nearly .012 inch... if you fit it up like I do with .003 gap.
Third... You're not factoring in the strike of the firing pin driving the case forward and giving the barrel a little more push. So, even if the feeler gauge reveals .012 inch gap... it doesn't guarantee that the same gap will be present when the gun fires. And then... when the next round goes in the chamber... if the case length is different... you have a completely different set of dynamics to consider.
Finally... I'm so tired of talkin' about this firing pin stop radius that I could scream. I'm startin' to wish I'd never mentioned it.
I know what it does... why it does it... and how it does it. I also know that its effect is magnified when the gun is in motion at full speed. I also know whast its effect is on the recoil characteristics of the gun...a nd I know that no two guns will respond in the same way. I know that... even the shooters who can't actually feel the difference... though the biggest percentage do... can see a difference in muzzle flip and reaquiring the target after the gun recoils. I don't really care how much difference there is and can be proven on paper. It's like paper ballistics. They don't tell the whole story. 1% or 2% or 22%. It just don't matter. All that matters is the end result. That being that th gun spreads out recoil from the spring over a little longer time frame... and that the slide strikes the impact abutment with less momentum and energy... and that makes a difference in the gun's shootability.
So... I ain't gonna argue the points any more.
Ya'll carry on, now. Hear?
Quote:
The 0.0125" lug face gap allows the pressurized case to accelerate the
slide back without the barrel before the gap is closed and the slide starts
dragging the barrel along with it.
What do you want to bet that in the initial nanosecond or three when this might be happening... it won't happen, because the case is expanded tight enough in the chamber that it can't push back on the slide independent of the barrel?
Don't want to make you scream, so no response necessary, amigo.
But I was using the average Gov't Issue (GI) gun in my previous post - nothing at all special & nothing at all fitted. So...
Quote:
First... Since the slide hits the barrel hood, it may not leave the full
.012 gap in the lugs.
In this average (I'd use the term "mid-spec", but I know it makes you cringe) GI gun, the slide's breechface never makes contact with the aft surface of the hood. When in battery, there's a gap of 0.006" between the hood & breechface and the barrel feet are held against the SS pin by contact between the slide's breechface guide blocks and the barrel's chamber face.
Quote:
Second... If the hood to breechface fit is tight... that gap doesn't
exist... or there's not nearly .012 inch... if you fit it up like I do with
.003 gap.
Again, the gun's an average GI M1911A1 with nothing fitted. This gun will have that 0.0125" gap.
Quote:
Third... You're not factoring in the strike of the firing pin driving the
case forward and giving the barrel a little more push.
But the gun's already in battery with the barrel feet against the SS pin. So the firing pin tapping the case forward against the stop shoulder (if it's not already there) cannot make the barrel go any farther forward.
Quote:
So, even if the feeler gauge reveals .012 inch gap... it doesn't guarantee
that the same gap will be present when the gun fires.
The only way that 0.0125" gap could change before the cartridge ignites would be if the slide were to move aft against the, say, 8 pounds of force supplied by the recoil spring. Not sure what, if anything, could cause that.
Sorry if I didn't make it clear the gun in the example was just a plain old "rattles when shaken" 1911.
Cheers
P.S.: As a bedtime treat, here's a pic of an FPS from a gun made in 1912 that I stumbled across while looking for that 12" barrel; http://www.coolgunsite.com/images/1...260/fplate2.jpg
Quote:
What do you want to bet that in the initial nanosecond or three when this
might be happening... it won't happen, because the case is expanded tight
enough in the chamber that it can't push back on the slide independent of the
barrel?
Hmm-m-m-m. Interesting!
For this to occur, seems like the gas pressure would have stick the case onto the chamber prior to the onset of bullet/case separation. Hmm-m-m-m.
Quote:
For this to occur, seems like the gas pressure would have stick the case
onto the chamber prior to the onset of bullet/case separation.
OBTURAAAAAAATION!!
Quote:
OBTURAAAAAAATION!!
Thanks - hadn't thought of that. Let's see.
The Missouri Bullet website http://www.missouribullet.com/technical.php gives this formula relating the optimum bullet hardness (for obturation) and pressure: Optimum BHN = CUP ÷ 1280. Kuhnhausen gives 19,900 CUP for GI Ball ammunition. So for GI ball pressures, BHN = 19,900 ÷ 1280 = 15.5, and that's about what my straight wheel weight cast bullet test.
The Lyman Cast Bullet handbook implies BHN = 100 for a jacketed bullet's jacket, but never having tested one myself lets say they're acutally only BHN50. Such a bullet would take CUP = BHN X 1280 = 50 X 1280 = 64,000 CUP to obturate if the jacket covered the base too. But if the soft lead core's exposed at the base I'd think the pressure needed to obturate it would be less, perhaps less enough to make obturation possible. Perhaps not.
Bercovitz's study indicates peak pressure occurs after 0.3" of bullet movement - when most bullets' bases are at the case mouth - and too late for an obturating bullet to enlarge the case.
But, a fairly soft, heavy lead bullet loaded pretty hot (to where it would begin to obturate while still in the case) might do it.
Maybe bullet obturation does play a role. Be nice to test and see.
Quote:
Gad, I hadn't even got close!
There's one more.
The bullet is a big part of the slide's delay due to frictional forward drag on the barrel.
The longer the barrel, the longer the bullet has to drag on it. The longer the bullet drags on it... the more it delays it. You could make the barrel so long that the slide wouldn't cycle.
Quote:
You could make the barrel so long that the slide wouldn't cycle.
Sounds logical - and fun too!
The longer barrel adds mass to the slide & barrel slowing down the show. Make the barrel long (massive) enough and cause so much delay that the link never quite makes it into tension to pull down and unlock/disengage the barrel.
The added mass of the longer barrel might even prevent the slide from flicking the hammer back out of the way - slowing down the show even more.
Instead of a "BANG" there's a little "poot" as the bullet departs the barrel and the recoil spring pushes the slide & barrel forward a little back to battery.
And there's no doubt a barrel so long the bullet never even gets out of it.
Gosh, I sure hope nobody ever figures out what those two barrel lengths are!
Quote:
The added mass of the longer barrel might even prevent the slide from
flicking the hammer back out of the way - slowing down the show even more.
A few years back, me an' this other fool made up a nifty little suppressor and threaded the endcap to match a nifty little barrel bushing that had an extension and threads.
The supressor weighed about 2 pounds. Worked right good... except for the fact that the pistol became a straight-pull bolt action. It wouldn't push the hammer to half cock.
Of course, the can disapeared about 25 years ago, and we haven't seen it since. I don't know who wound up with it... and I don't wanna know. I do know that it was quieter than a Sionics unit that was on the end of a legal select- fire Mac-10 that a doctor let us play with one fine Sunday afternoon.
Quote:
The added mass of the longer barrel might even prevent the slide from
flicking the hammer back out of the way - slowing down the show even more.
Instead of a "BANG" there's a little "poot" as the bullet departs the barrel and the recoil spring pushes the slide & barrel forward a little back to battery.
Proven.
That's about what happened when I tried to prototype my .380 Auto 1911 project by shooting .380 ammo out of a Government length Super .38 barrel. It went "bang" (small 'b' - no exclamation mark) but it wouldn't cycle te slide, even with the lightest spring I had on hand cut several coils shorter. Decent accuracy, but I don't think there's much market for single-shot 1911s in .380 Auto caliber.
While it's all greek to me, I haven't seen anyone torque about talk. I mean talk about torque.
Quote:
While it's all greek to me, I haven't seen anyone torque about talk. I mean
talk about torque.
If you're talking about the gun twisting about the barrel's axis, search for a post within the last month or so by Log Man.
He reported some very interesting observations.
Log Man's barrel twist experiment results are in Post #8 of this thread: http://forum.m1911.org/showthread.p...highlight=twist
All by itself, a 1911 with a normal/usual left hand twist barrel will see the bullet begin to rotate counter-clockwise as it enters the rifling. The gun's reaction to this rotation would be a clockwise rotation. A clockwise rotation about the barrel axis would tend to make the grip also rotate clockwise or out of the palm of a right handed shooter.
But because the bullet is rotating as it goes down the barrel, any displacement of the bullet from its initial line of travel would (I think) result in some twisting of the gun in some other direction due to gyroscopic affects. This displacement would come from recoil.
At least that's how I understand it. All my 1911 barrels have left hand twists, and I'm not usually aware of any twisting when shooting. However...
...once in a while I'll be doing some experimenting or modifying, fire a shot, and the gun feels like it was almost torn from my right hand grip. The next shot's back to normal - little or no perceived twisting.
And I've absolutely no idea why a 1911 would behave like that.
Perhaps someone else knows.
I wish I could recall my college robotics and physics, because I thought there was something in there about a lift perpendicular to the axis of rotation (based on a right-hand rule).
It's the same thing that causes a piece of play-do being rolled out into a noodle to flip up at the end, or for a tornado to form in the vertical axis when two air masses are causing roll in the horizontal.
So I just figured you gentlemen would account for some sort of torque-related lift force in your calculations. (I am way out of my league here, and I know it!)
I don't think there's any gyroscopic affect on a rotating body until there's some perturbation of it's rotational axis. Note the "...I don't think..." part.
Read somewhere about some lifting effect on a bullet once it leaves the barrel, but didn't follow it too closely. And I think you're interested in what happens while the bullet's still in the barrel, right?
Maybe somebody with some smarts will chime in and shed some light.
"Gyroscopic Precession" is the term. It's indeed the reaction to perturbation. Those who ride motorcycles will know that to turn left, that is to "bank" left, a little pressure forward on the left handlebar will cause the front wheel to tilt to the left, setting up the turn to the left. So turning the handlebar right will turn the motorcycle left (by causing the front wheel to "flop" to the left).
As for the effect on the barrel from the spinning bullet, I'm inclined to think that the barrel would try to rotate the same direction as the bullet, due to the friction of the bullet "dragging" the barrel around. I'm fairly certain that when you "slug" a bore with a soft bullet, the bullet tries to drag the barrel with it, rather than pushing the barrel the other way .
Quote:
I'm fairly certain that when you "slug" a bore with a soft bullet, the
bullet tries to drag the barrel with it, rather than pushing the barrel the
other way.
Well,I sat here looking down my GI barrel (left hand twist, land on top curving around to the left, forcing the bullet to rotate counter clockwise) and thought the barrel would twist clockwise when something was poked down it. But I've thought wrong plenty of times in the past, so...
...off to shop with barrel, glued a cork on the end of a dowel, chucked the dowel/cork up in a drill press and pushed the cork into the rifling (drill press not running).
As I'd suspected, the barrel rotated clockwise in reaction to the non- rotating cork being shoved into it.
I'd also suspect if both were free to rotate the cork/bullet would go counter clockwise and the barrel would go clockwise. Action & reaction?
Unless I've goofed up badly I think that pretty much debunks any notion that JMB went for a left hand twist so the gun would twist more into the palm of a right handed shooter. Mine don't. Saw a forum post somewhere that J. Hatcher attributed the left hand twist to early 1911 barrels being made in England on machinery already set up for a left hand twist - and JMB simply stuck with that handedness.
Seriously dumb series of math errors from earlier corrected below, you are strongly encouraged to check my work:
Making some simplifying assumptions*, the torque necessary to accelerate a 230gr bullet to full speed (an astounding 64,000 rpm) going down a 5" barrel is about .7 N-m, or about .43 ft-lbs. And that lasts for about .001 sec until the bullet leaves. You're going to have a hard time feeling that through a 2lb lump of steel, especially with the recoil coming back at you.
* Assumptions:
Bullet is conical and homogeneous (real ogive will result in slightly higher force, copper jacket will result in slightly lower force)
Final speed is 800 fps
Twist is 1 in 9"
Acceleration occurs uniformly down barrel (known to be false, but a heck of a lot easier than trying to work it out).
Quote:
Twist is 1 in 9"
And the usual twist is 1 in 16", so I think this would see a significant reduction in your results.
I'd often wondered if the real violent clockwise twists I'd encountered a few years ago were the results of a bullet in my powder-puff handloads failing to get out of the barrel before the onset of linkdown. Slide & barrel lugs still a little pressure locked, link tugs on back of barrel, barrel doesn't want to disengage real easily, sudden tug on the barrel causes the spinning bullet to do some gyroscopic gyrations, barrel does same, slide & frame do same, I mutter "Dang! What was that?".
But, I have no real idea what caused that to happen - except that it was quite a violent clockwise twisting (and probably not a spastic muscle contraction ).
OK, so with the correct twist rate (was browsing the Brownell's AR-15 catalog last night), the numbers got to .4 N-m or .24 ft-lbs, for .001 second.
But, I should point out that I caught several significant errors in my initial calculation, so anybody who is so inclined should check my work to make sure I'm not completely off base here.
Quote:
I'd also suspect if both were free to rotate the cork/bullet would go
counter clockwise and the barrel would go clockwise. Action & reaction?
Niemi, you startled me for a minute, until I realized that the cork was non- rotating. Alas, I fear this demonstration is not relevant. You have to do it with a freely rotating cork (and a barrel arranged so that you can get a feel for the barrel's reaction).
Quote:
Niemi, you startled me for a minute, until I realized that the cork was
non-rotating. Alas, I fear this demonstration is not relevant. You have to do
it with a freely rotating cork (and a barrel arranged so that you can get a
feel for the barrel's reaction).
Wasn't sure if it really made any difference, so...
...back to the shop, cork/dowel retrieved from trash, put empty 45 case over end of dowel to allow the cork (bullet) to rotate as the case was pushed down and here's the results:

Here's another way to imagine it: pretend you have a barrel with only an inch-long section of left hand twist land about half way down. This section's on the top of the barrel, so it veers off to the left from its closest to its farthest extent when viewed from the chamber.
Fire a round and the bullet initially chugs down the smooth barrel without any rotation at all - until it hits the little land section. The top of the bullet will be deflected to the left (go CCW) and the top of the barrel will get deflected to the right (go CW). Action & reaction.
Unless the cork I used is haunted, methinks that's what happens in a real gun with a left hand twist barrel.
Quote:
...anybody who is so inclined should check my work to make sure I'm not
completely off base here.
And that's not Mrs. Niemi's little boy! No clue how to do that sort of stuff. But...
...I should add that even on the very rare occasions when I do shoot GI Ball (in that gun that twisted violently a few times) I feel absolutely no twisting motion or "torquiness".
Like Log Man reported, what twisting a person does feel is probably a result of how the bones, muscles & tendons in the arm react to the straight back recoil.
Action/reaction rotational force/counter-rotational force. JMB put that force against the meat of the right handed shooter's hand,
Quote:
Action/reaction rotational force/counter-rotational force. JMB put that
force against the meat of the right handed shooter's hand
Except that he didn't; a left-hand twist tends to cause an opposing reaction twist, which pulls the grip to the left, away from the right-handed shooters palm. This is shown quite clearly in Niemi's picture; the barrel feet rotate the same way the grip would. Fortunately the impulse is miniscule.
I just checked my RIA widebody, and it somewhat interestingly has a right- hand twist in an otherwise standard barrel.
Quote:
...I should add that even on the very rare occasions when I do shoot GI
Ball (in that gun that twisted violently a few times) I feel absolutely no
twisting motion or "torquiness".
And when that gun with the left hand twist barrel twisted violently, the gun felt like it nearly twisted itself out of my right handed (and one handed) grip by twisting clockwise.
One right handed Bullseye shooter has a Clark (I think) 1911 with a right hand twist barrel and I observed that gun twisting counter clockwise into the palm of his hand.
Taking Log Man's post (#8 in this thread http://forum.m1911.org/showthread.p...highlight=twist ) a bit further, maybe it's even possible for one right handed shooter to have a gun twist one way and another right handed shooter have that same gun twist the other way. Log surmised the twist direction had something to do with the structure of the shooting arm and different shooters may have different arm structures.
Beats me. I'm no fizzyologist!
I think somewhere earlier in this thread (or was it another? So much information, so little time...) that the consruction & function of "long slide" M1911s was introduced.
Jim Hoag built 6" and 8" long slides, and one 11" just because he could. At the time (1979) he averred that he would not build another for less than three grand. Thirty years ago you could almost buy a new car for three grand.
The details are in the September-October 1979 issue of The American Handgunner, now available in PDF from their web site. Warning: it's about a 40MB file.
Thanx for link to the Hoag article and his 11" 1911. Let's see, today's equivalent of $3,000 in 1979 would be about $8,900 according to this: http://data.bls.gov/cgi-bin/cpicalc.pl .
Couldn't afford the $3,000 either!
Hey Walt, if you are a movie buff check-out Michael Mann's Thief from 1981, James Caan carries a 6" Hoag. It's also the only movie I know of that gives credit to a gunsmith.
There's another book around here that has more on the 11" Hoag. I'll see if I can find it.
Found it!

Wonder how much spring wire Hoag used up before finally winding one that worked!
Quote:
Wonder how much spring wire Hoag used up before finally winding one that
worked!
Call him and ask!
James Hoag
8523 Canoga
Canoga Park, CA 91304-2662
818-998-1510